Rational and Irrational Numbers

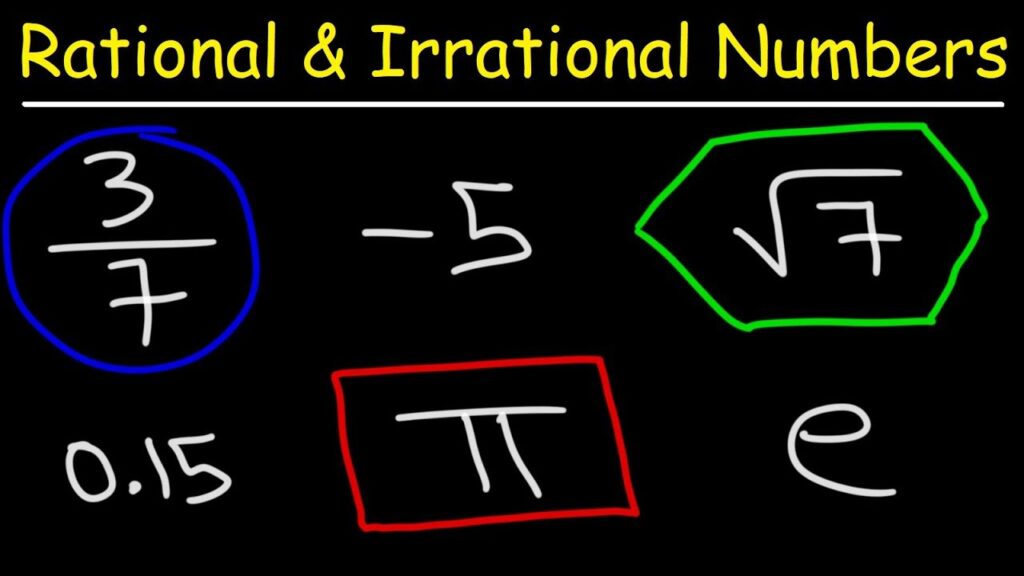
Rational Numbers and Irrational numbers are both real numbers; however, they are different in their properties. Rational numbers can be expressed by way of a a ratio. On the other hand, irrational numbers are only expressed in the form of a fraction. Let us understand this in a better way with a few examples.

Rational number

It is a number that can be depicted in the P/Q form. P and Q both are integers, also Q ≠ 0. However, an irrational number is not written in simple fractions. For example, ⅔ is a rational number, whereas √4 is referred to as an irrational number.

Let’s know more with examples. Also, we will learn the differences between rational and irrational numbers.

What are Rational numbers?

They are numbers that are illustrated as fractions, and they are positive and negative and also zero. A rational number is written in the form of p/q. Here Q ≠ 0.

The term rational originates from the term ‘ratio’. It means comparing two and more integer numbers, and it is called a fraction. Simply put, it is two integers ratio.

• Example 1

4/2 is one such rational number, which means 3 is an integer divided by the integer 2.

• Example 2

Is 9 a rational number?

9 is a rational number as it fulfils the criteria of a rational number. 9 is expressed in the form of a ratio like 9/1. The denominator is none zero.

What are Irrational Numbers?

The numbers that are not rational numbers are referred to as irrational numbers. Let us show you how an irrational number can be written in decimal but not in fraction form. This means it is not written as two integers ratio.

After a decimal point, an Irrational number has infinite digits. Here is an example, √8=2.82828.

How Do You Classify Rational Numbers and Irrational Numbers?

Let’s learn how to specify rational numbers and irrational numbers established in the few examples given here. According to the definition, rational numbers contain all integers, repeating decimals and fractions. Each rational number can be written in the p/q form, where p and q are both integers.

So how are Rational and Irrational numbers different? Rational numbers are repeating decimals, finite, whereas an irrational number is non-repeating and infinite.

Venn Diagram

Here, the image demonstrates the Venn diagram for rational numbers and irrational numbers. They fall in the category of real numbers.

Differences Between Rational Numbers and Irrational Numbers

Rational Numbers

• These are illustrated in the form of a ratio. The numerator and denominator are both whole numbers.
• It contains perfect squares.
• The expansion of decimals for a rational number achieves finite decimals or recurring decimals.

Irrational Numbers

• It is impossible to illustrate irrational numbers in the form of fractions or the form of ratios of integers.
• It contains surds
• In irrational numbers, non-recurring and non-terminating numbers are achieved.

Some illustrations of Rational numbers and Irrational numbers are shown here.

Rational Numbers Examples

• Number 8 is written as 8/1, where 8 and 1 are both integers.
• 25 is written as ¼, or 5/20 or 10/40, or in the terminating decimal forms.
• √16 is also a rational number since it is simplified to 4 and expressed as 4/1.
• 999999 is in the form of recurring decimals. It is called a rational number.

Irrational Numbers Examples

Likewise, as we already mentioned that an irrational number could not be illustrated in a fraction form or ratio form. Let’s infer the theories with some examples.

• 4/0 is called an irrational number; the denominator is zero.
• π is called an irrational number. Its value is 3.143. It is a nonrepeating and continuous number.
• √3 is also called an irrational number since it can’t be simplified.
• 313113111…is called a rational number because it is non-terminating and non-recurring.

There are several examples other than the above-given ones that differentiate rational and irrational numbers.

Some other illustrations of rational numbers and irrational numbers are

Rational numbers examples are 1/3, 5/4, 9/4, 3/100, etc.

Irrational numbers examples are √3, √5, π(Pi).

Interesting fact

When decimal numbers are illustrated by bars, are they referred to as rational numbers or irrational numbers?

Decimal numbers with a bar represent a rational number since the number after the decimal point is repeated.

Rational Numbers and Irrational Numbers Properties

Listed below are a few rules founded on arithmetic operations like multiplication and addition executed on rational and irrational numbers.

Rule #1

When you add two rational numbers, it is also a rational number.

For example, ⅓ + ¼ = (4+3)/12 = 7/12

Rule #2

When two rational numbers are multiplied, the number that is obtained is also rational.

For example 1/3 x 1/3 = 1/9

Rule #3

When you add two irrational numbers, the answer is not invariably an irrational number.

For example, √3 + √3 = 2√3 is an irrational number.

2 + 3√5 (-3√5) = 2  is a rational number

Rule #4

When two irrational numbers are multiplied, the result is not invariably an irrational number.

Example: √4 x √3 = √12. A result is an Irrational Number.

√3 x √3 = √9 = 3. The answer is Rational Number.

Conclusion

Therefore, we can conclude that Rational numbers are depicted in the ratio form, P/Q, where Q≠0. Irrational numbers are depicted in the form of a fraction. Both these numbers are Real Numbers, and they are represented in the form of a number line.